Rock wool, glass wool, hemp - which material is best suited for absorbers?

Are you currently dealing with the question of how you can improve the acoustics in your listening room? If you are like me, then the question of the size of the absorbers should be resolved relatively quickly. Because most suppliers of mineral wool offer similar sizes in the area of ​​120cm x 60cm (4’ x 2’).

At Absorber Depth, you are probably struggling with two goals: deep enough to properly absorb bass, but small enough not to waste too much space in the room. Because we all have walls around us somewhere that we cannot move.

And ultimately, at the latest when you ask about the right material for the interior of your absorber, you will stumble - at least that's how I felt. There are many examples of how to build absorbers yourself for the recording studio or home theater. Simply copying it would be an option, hoping that people would think of something when choosing it. And every mineral wool absorbs better than a bare wall! So the chances are good that you will achieve an improvement in any case.

Ultimately, of course, remodeling your room will cost time and money. In the best case, the values ​​such as absorber depth and absorption properties should fit your goals.

So that the values ​​are in the right range even when starting up for the first time, I would like to give you a little guide today for choosing the right insulation wool.

Rock wool or glass wool - which absorbs better?

To shorten this striking question: Both can be used excellently and are also used by the most renowned recording studios and acousticians worldwide. There are also a number of other substances such as hemp, Basotect or Caruso Iso Bond, all of which have very good absorption. Much more important than the question of the material itself is a very important key figure. No other size can better determine how large the (frequency-dependent) absorption will be later:

The specific flow resistivity

Put simply, this value describes how much the speed of the air vibration decreases when passing through the material. So it is measured in front of and behind the absorber material. The lighter the material, the more it arrives after crossing. And the thicker the material, the less it gets through, but the more it is reflected. The art for us later will be to find a material that is heavy enough to absorb as much as possible, yet light enough that the sound is not reflected halfway through the material and the last cm of the absorber is no longer at all reached.

There are occasionally two values ​​in data sheets. One is the flow resistance specifically related to this material thickness (for example 5 cm). It has the unit Pa * s / m. Since each manufacturer has different thicknesses in its range and we still want to determine which thickness is suitable for us, this value alone does not help us.

The second value is the length-specific flow resistance at which the previous value is divided by the material thickness. It is therefore purely dependent on the material and no longer on the thickness that was used for the measurement. It can be recognized by the unit Pa * s / m2.

With common sense it can be guessed that there is a connection between the density, i.e. the specific weight of the material, and the flow resistivity. In order to get an overview of the areas in which this value is located, I have worked through some products of common mineral wool and have written down the flow resistance and density.

Material	Manufacturer	Product	Flow Resistivity	Density		Price per m³
Glasswool	Bradford	Acoustiguard	5800 Pa*s/m²	14 kg/m³	0.9 lbs/ft³	45 EUR	50 USD
Glasswool	Bradford	Absorb Black	8000 Pa*s/m²	18 kg/m³	1.1 lbs/ft³
Glasswool	Bradford	Ultratel	25000 Pa*s/m²	48 kg/m³	3.0 lbs/ft³
Glasswool	Isover	TP-1	5000 Pa*s/m²	13 kg/m³	0.8 lbs/ft³	35 EUR	39 USD
Glasswool	Isover	SSP 1	11000 Pa*s/m²	20 kg/m³	1.3 lbs/ft³	133 EUR	146 USD
Glasswool	Isover	E60 S	22000 Pa*s/m²	30 kg/m³	1.9 lbs/ft³
Glasswool	Isover	PB A 31	37000 Pa*s/m²	50 kg/m³	3.1 lbs/ft³
Glasswool	Knauf	TP 120A Ecose	11000 Pa*s/m²	20 kg/m³	1.3 lbs/ft³	350 EUR	385 USD
Glasswool	Owens Corning	701	14000 Pa*s/m²	24 kg/m³	1.5 lbs/ft³
Glasswool	Owens Corning	Fire & Sound Guard	20000 Pa*s/m²	40 kg/m³	2.5 lbs/ft³
Glasswool	Owens Corning	703	27000 Pa*s/m²	48 kg/m³	3.0 lbs/ft³
Rock wool	Knauf	KR S	9500 Pa*s/m²	32 kg/m³	2.0 lbs/ft³
Rock wool	Knauf	KR SK	10300 Pa*s/m²	40 kg/m³	2.5 lbs/ft³
Rock wool	Knauf	KR L	13800 Pa*s/m²	50 kg/m³	3.1 lbs/ft³
Rock wool	Knauf	KR M	14300 Pa*s/m²	60 kg/m³	3.8 lbs/ft³
Rock wool	Rockwool	Termarock 30	7000 Pa*s/m²	30 kg/m³	1.9 lbs/ft³	95 EUR	105 USD
Rock wool	Rockwool	Sonorock 035	8000 Pa*s/m²	23 kg/m³	1.4 lbs/ft³	85 EUR	94 USD
Rock wool	Rockwool	201	10000 Pa*s/m²	35 kg/m³	2.2 lbs/ft³
Rock wool	Rockwool	Termarock 40	10000 Pa*s/m²	40 kg/m³	2.5 lbs/ft³	95 EUR	105 USD
Rock wool	Rockwool	ProRox SL 920 = RWA45	10000 Pa*s/m²	40 kg/m³	2.5 lbs/ft³	80 EUR	94 USD
Rock wool	Rockwool	Airrock LD	10800 Pa*s/m²	40 kg/m³	2.5 lbs/ft³	70 EUR	77 USD
Rock wool	Rockwool	Airrock ND	14400 Pa*s/m²	50 kg/m³	3.1 lbs/ft³	80 EUR	88 USD
Rock wool	Rockwool	225	16000 Pa*s/m²	46 kg/m³	2.9 lbs/ft³
Rock wool	Rockwool	Termarock 50	16000 Pa*s/m²	50 kg/m³	3.1 lbs/ft³	120 EUR	132 USD
Rock wool	Rockwool	Airrock HD	20700 Pa*s/m²	70 kg/m³	4.4 lbs/ft³
Rock wool	Rockwool	221	22000 Pa*s/m²	55 kg/m³	3.4 lbs/ft³
Rock wool	Rockwool	ProRox SL 930 = RW3	28000 Pa*s/m²	60 kg/m³	3.8 lbs/ft³	105 EUR	116 USD
Rock wool	Rockwool	ProRox SL 950 = RW4	42000 Pa*s/m²	80 kg/m³	5.0 lbs/ft³	110 EUR	121 USD
Rock wool	Rockwool	Termarock 100	43000 Pa*s/m²	100 kg/m³	6.3 lbs/ft³	260 EUR	286 USD
Rock wool	Rockwool	ProRox SL 960 = RW5	50000 Pa*s/m²	100 kg/m³	6.3 lbs/ft³
Polyester	Caruso	Iso Bond WLG 045	3000 Pa*s/m²	15 kg/m³	0.9 lbs/ft³	220 EUR	242 USD
Polyester	Caruso	Iso Bond WLG 040	5000 Pa*s/m²	20 kg/m³	1.3 lbs/ft³	405 EUR	446 USD
Polyester	Caruso	Iso Bond WLG 035	10000 Pa*s/m²	40 kg/m³	2.5 lbs/ft³	950 EUR	1045 USD
Melamine resin	BASF	Basotect	12000 Pa*s/m²	9 kg/m³	0.6 lbs/ft³	950 EUR	1045 USD
Hemp wool	Thermo Natur	Thermo Hemp Premium	3000 Pa*s/m²	35 kg/m³	2.2 lbs/ft³	105 EUR	116 USD
Hemp wool	AWB	Hemp	1200 Pa*s/m²	50 kg/m³	3.1 lbs/ft³
Sheep wool	Deamwool	Schafwolle	290 Pa*s/m²	13 kg/m³	0.8 lbs/ft³

The ultimate goal of this article is that you find the right material for you and your goals. Therefore, we will return to this table later. As a rough guide, I wrote down the prices (converted per cubic meter) as I could find them on the Internet. Of course, this is subject to some fluctuations, depending on the country and possibly plus shipping. But I think it is important to get a feel for the price differences between acoustically similar materials.

The relationship between weight and flow resistivity is most clearly shown in a graphic. I have entered each of the aforementioned products with their two values (left is kg/m³, right is lbs/ft³):

On the one hand you can see that there are certain fluctuations and the values ​​should only be used as a rough guide. Nevertheless, a certain linearity can be seen for each material type. Any flow resistivity can be achieved with almost all materials. Depending on the type, this requires a different material density.

The most important finding when comparing glass wool vs. rock wool: rock wool must be about 50% heavier than glass wool to achieve the same flow resistivity. For example, we achieve the value of 10000 Pa * s / m² with 35-40kg / m³ rock wool, or with 20kg / m³ glass wool.

Caruso Iso Bond, on the other hand, is very similar to rock wool. The flow resistivity of 10000 Pa * s / m² can be achieved with both materials with a material density of 40kg / m².

But now finally to the actual questions of today's article. Let's start with:

Which flow resistance is optimal for my absorber depth?

With this question you can already see how I would approach the material selection: first we determine the correct range for the flow resistivity. And then we use the material table to see which material with which weight can be used to achieve this flow resistivity.

And don't worry if you are still undecided about the absorber depth. After the following examples, we will once again address the question of how thick the material may be at the various points.

Tools to simulate the degree of absorption

Since it is very time-consuming to impossible to acoustically measure all materials and all combinations at home, I have come to appreciate a (free!) Online tool. Of course, every simulation is only an approximation. But to get a feeling for the effects of different absorber depths and flow resistances, I know of no better and easier way than this calculator: http://www.acousticmodelling.com/porous.php

There are some limitations to keep in mind when using this tool. For one thing, I assumed an angle of 0 degrees for the simulation, i.e. we assume a vertical angle when the sound hits the wall. This is the case, for example, when we think of the wall behind the speakers. In practice, this angle changes somewhat for absorbers on the side walls, depending on how large the width of the room is and how large the listening distance is. In my experience, the 0 degree bends are the “worst”, i.e. a low degree of absorption is displayed. If we can increase the angle in practice, the values ​​should always be better than the simulation for 0 degrees, since the sound travels more through the absorber at an oblique angle of incidence and is therefore better damped.

The tool only calculates with the absorber depth and the flow resistance. There is no way that the material density is taken into account. In this respect, the values ​​are to be treated with caution and can differ slightly in reality.

Another assumption of the tool is the use of an infinitely large absorber wall. The output values ​​are only achieved if a sufficient number of absorbers are placed side by side without gaps. I think it makes sense that we cannot conquer a 100 Hz wave (with a wavelength of 3.40 m) with a single absorber in the size of 1.20 m x 0.60 m. At low frequencies, we should be aware that we have to apply large areas. At high frequencies, i.e. if the absorber is larger than the wavelength (for example, 1 kHz has a wavelength of 34 cm), we can already achieve good absorption with a single absorber.

For the sake of completeness, the technical parameters that I used for my curves: air temperature: 20 degrees Celsius, air pressure: 101325 Pa, Angle of Incidence: 0 degrees, Porous Model: Allard and Champoux (1992).

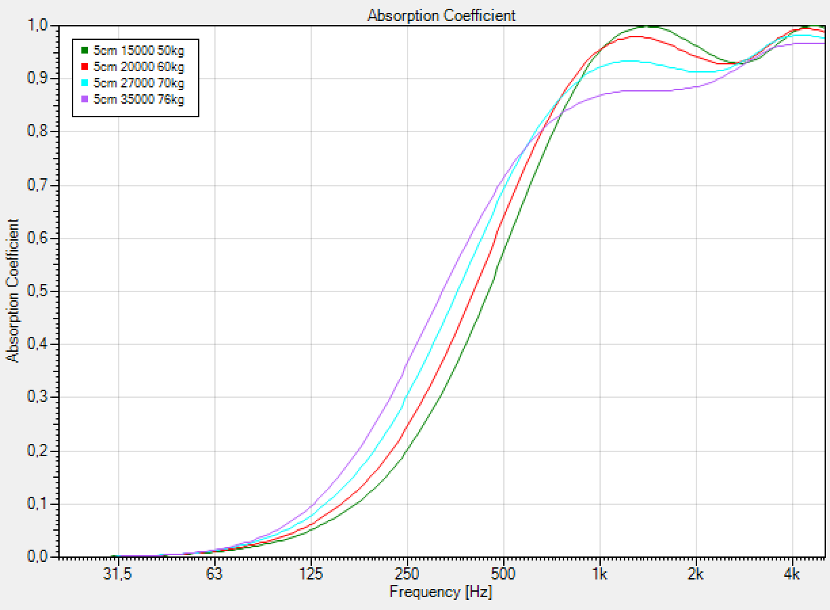
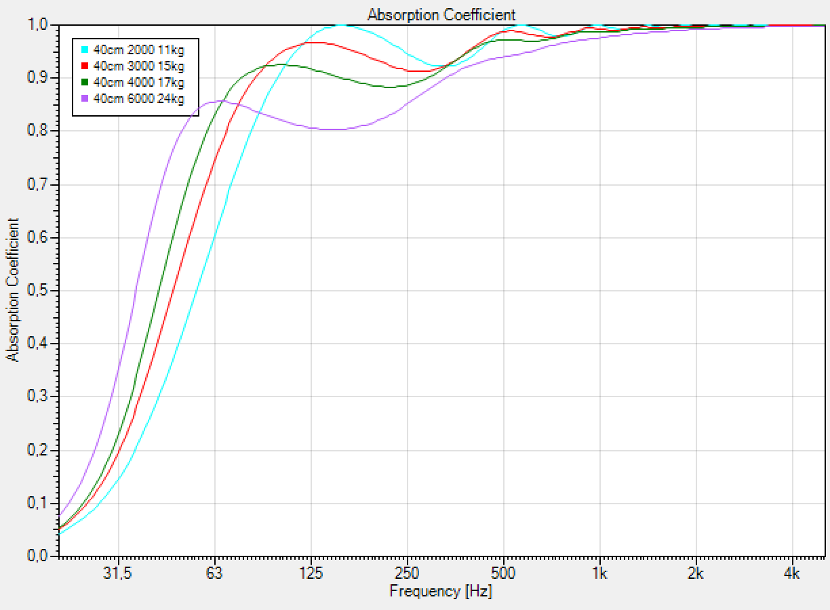
To classify how well the curves of the free tool compare with professional software, I performed the same simulation with the Soundflow software from AFMG (second graphic). The specific weight is also taken into account in the calculation. I used the density of the respective rock wool here. At low frequencies, the degree of absorption is somewhat higher compared to the calculation without weight.

With Soundflow I also used an angle of incidence of 0 degrees and an infinitely large area as parameters. Bies was used as a model.

Examples of different absorber depths

Using the following examples, I would like to give you a small guideline to find a reasonable flow resistance.

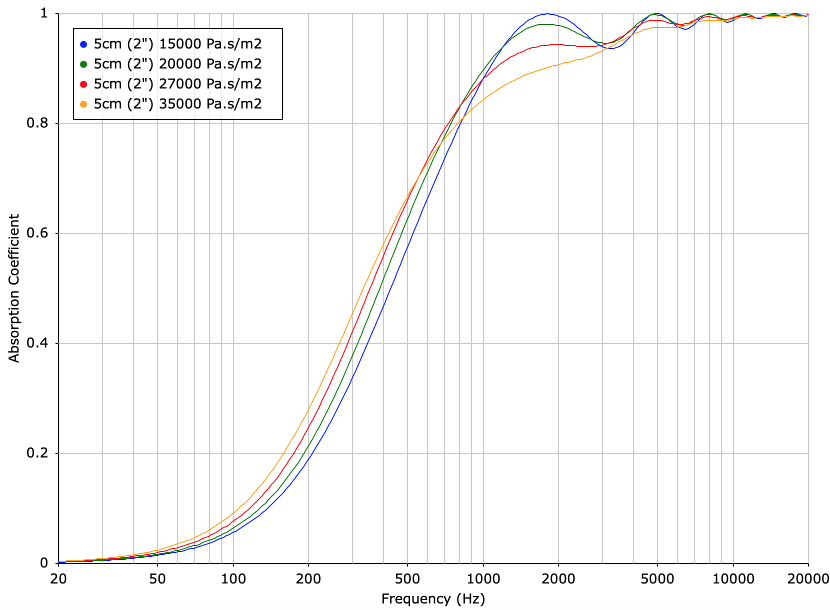
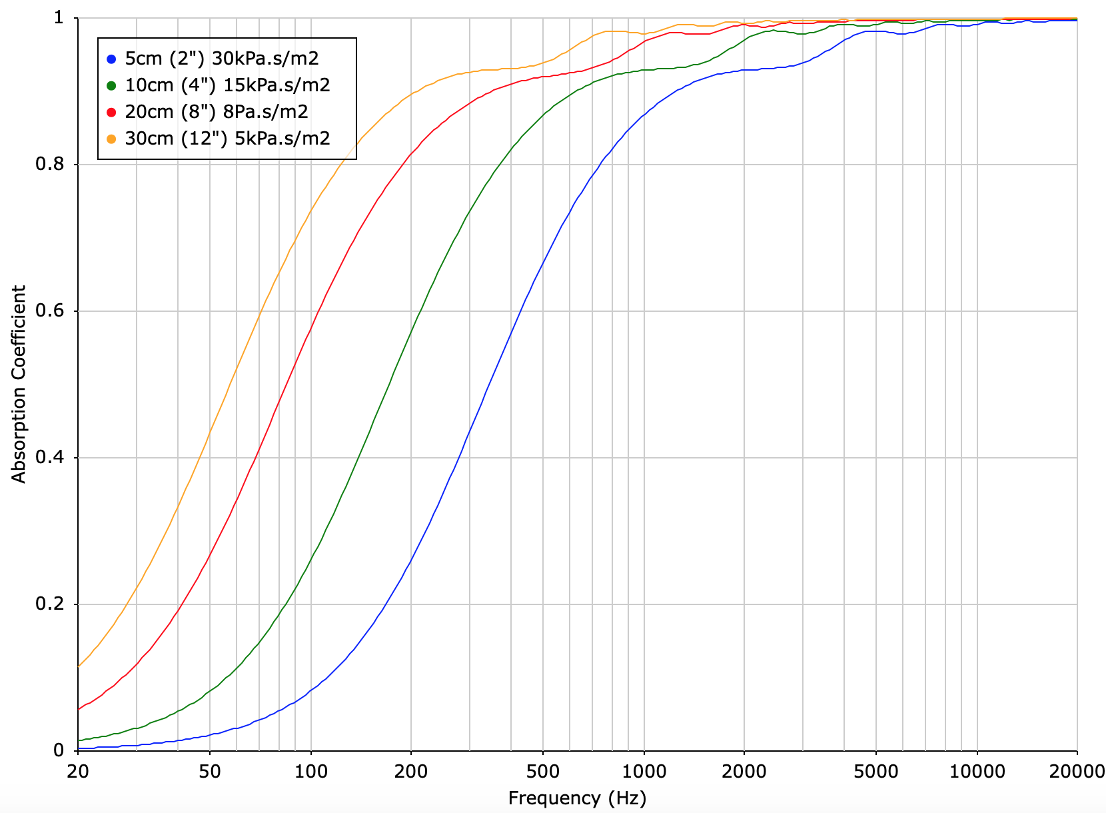
5cm without gap

As you can imagine, at a shallow depth of 5cm there are limits to the effectiveness in the lower frequency range. No matter which flow resistance we use, the damping is hardly available below 200Hz.

Nevertheless, a high absorption can be produced from 400 Hz. This depth is therefore always suitable for reducing early reflections on the side walls or on the ceiling. Since it strongly dampens the highs, but hardly changes anything in the bass, this type of absorber should only be used at specific points and should not be used on a large scale.

I see the target value for the flow resistance as about 30,000 Pa * s / m², so that a certain absorption is also achieved in the lower frequency range.

5cm (2”) with 5cm (2”) wall distance

With the same amount of material and thus costs, we can shift the absorption curve down by about an octave by adding an additional wall distance. Whenever it is technically possible, it is worthwhile to provide some space.

Based on the graphic, I would aim for a flow resistance of around 25000 Pa * s / m². Higher values have a slightly better absorption in the lows, but lose absorption above 300 Hz.

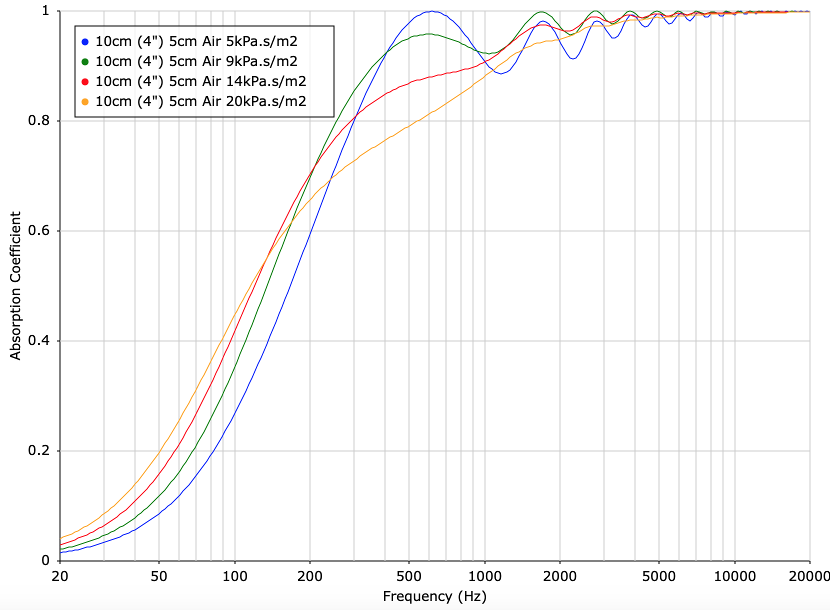
10cm (4”) without gap

It is of course even better than a 5cm absorber with 5cm wall distance if we fill the full 10cm with absorption material. As you can see from the four example values I selected, I see 25000 Pa * s / m² as the upper reasonable value for the flow resistance. As the best compromise, I would choose a material in the range of 15000 Pa * s / m².

Basotect is in this range both in terms of flow resistivity and material thickness. If it is rock wool, then you do very well with 45-50kg / m³, with glass wool with 25-30kg / m³.

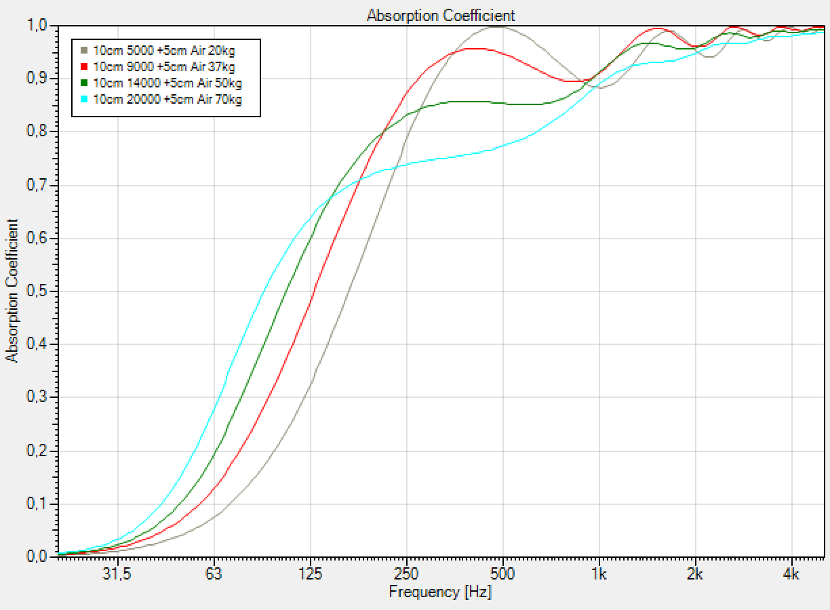
10cm (4”) with 5cm (2”) gap

Even with an absorber depth of 10cm, the effectiveness can easily be extended by a few cm distance from the wall. An absorption factor of 0.5 was possible without a wall clearance at around 200Hz. With a distance of 5cm we reach it at 130Hz.

In my view, the target value for the flow resistivity is around 14000 Pa * s / m².

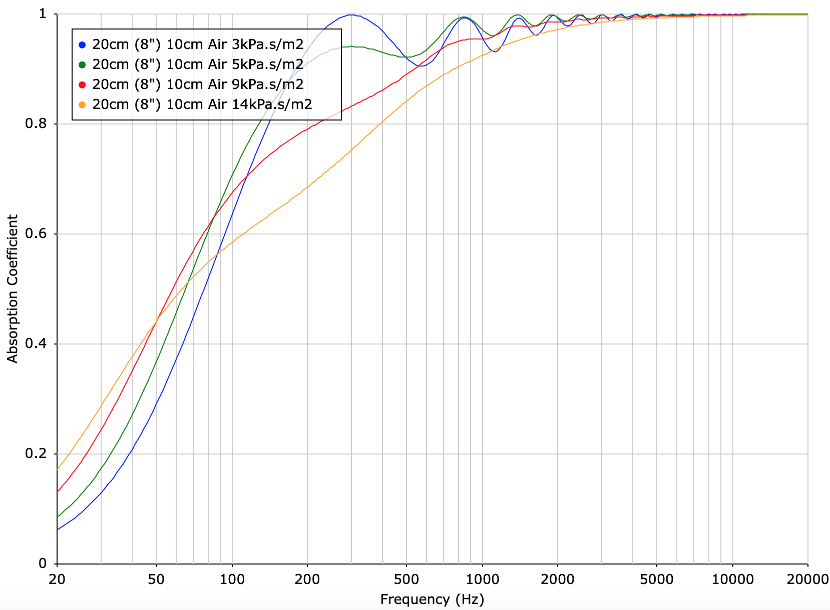
20cm (8”) without gap

From a material thickness of 20cm, we can slowly speak of broadband absorbers. Because even below 100 Hz we achieve a noticeable absorption. I see a reasonable flow resistivity at around 8000 Pa * s / m². Thus, for stone wool we have a density in the range of 30-35kg / m³, for glass wool around 15-20kg / m³.

20cm (8”) with 10cm (4”) gap

Even if our 20cm absorber depth has remained, the curve extends significantly further down to low frequencies due to an additional wall distance of 10cm. We now reach the absorption level of 0.5 not only at 90 Hz, but already at around 60 Hz.

I personally would also adjust the flow resistivity with an additional wall clearance and choose a slightly lighter material. Since I want to operate my wall absorbers pretty exactly in this constellation (20cm depth and 10cm wall distance), I took a closer look at this case. I would choose a flow resistivity of 6000 Pa * s / m².

Since I want to try Caruso Iso Bond because of the cleaner processing, I have already ordered some packages with WLG 040. There are not exactly 6000 Pa * s / m², but the 5000 Pa * s / m² that the manufacturer promises are close enough. This lower value also has advantages if I want to increase the absorber depth to 30cm on my back wall.

The difference between my current absorbers with 14000 Pa * s / m² rock wool and the 5000 Pa * s / m² Iso Bond will not be huge. Because in addition to the flow resistivity, it is ultimately of great importance for the overall effect how many absorbers I use and where I position them.

But I'm still curious! I will try to find out to what extent the forecast of this calculator is also noticeable in measurement in the real living room by comparing absorbers of the same size.

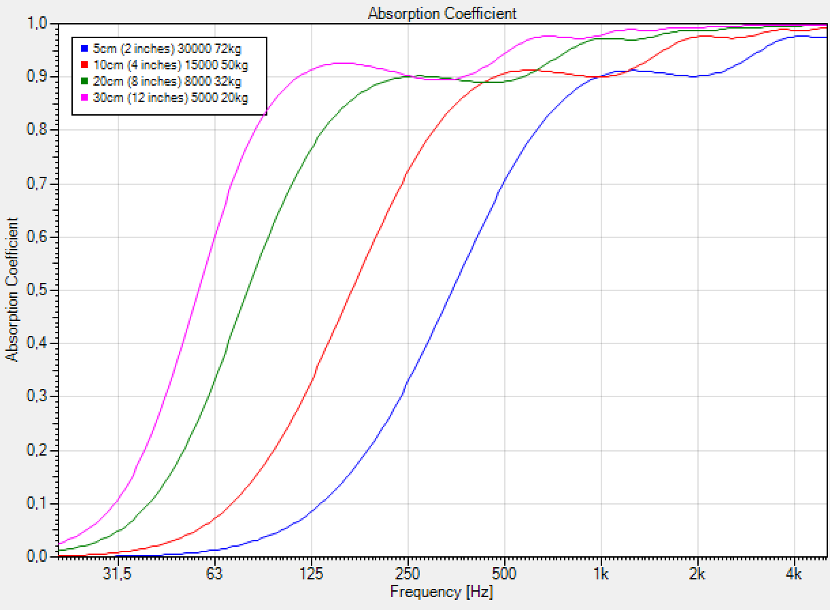
30cm without wall distance

If you find space in your room to install a 30cm deep absorber, you can count yourself lucky! For me, this doesn't quite work on the narrow side. At the end of the long wall, it could soon go in this direction. After all, who doesn't like crisp bass and a balanced frequency response even below 50Hz ?!

As you can see from the graphic, such an absorber depth is only worthwhile for very light materials. The orange curve with 11000 Pa * s / m² is already much weaker in the 100-500Hz range than all other curves. I would consider 5000 Pa * s / m² as optimal at a depth of 30cm. With my Caruso Iso Bond WLG 040, I am well prepared to set up such an absorber.

In the rock wool sector this value can be produced with a density of 15-20kg / m³, with glass wool with around 10-15kg / m³.

40cm without gap

Welcome to the area of professional recording studios! Even deep room modes can be weakened with 40cm mineral wool. As you can imagine, we have to use a lighter material than before. 6000 Pa * s / m² are almost a bit too dense. A flow resistance of about 3500 Pa * s / m² would be better. And so we have now reached a value that can actually be achieved with hemp wool.

How deep should my absorber be?

Based on the concrete examples, you have now got a feeling for the changes in absorption with increasing absorber depth. If there is enough space in your room, then of course you can achieve the best results with 40cm. Professional mastering studios, which want to hear every frequency equally well in the low frequency range and have to make decisions, are in this range with their absorption, sometimes even higher. As we have seen, such a thickness is only worthwhile if we can also procure a material that has a correspondingly low flow resistivity.

Money almost plays a subordinate role in this decision, because mineral wool is usually cheaper the lighter it is. Only the wooden construction becomes more expensive with larger absorbers. In most cases, the available space will ask us to deviate somewhat from the ideal. And if we don't want to open a mastering studio, there is nothing to be said against using far less.

Ultimately, it is a question of the goal, i.e. how short the reverberation should be in the low frequency range and how many dB the frequency response may fluctuate. Music can also be heard very well in a completely untreated room. However, due to the longer reverberation and the pronounced room modes, it is less defined and balanced than in a well-treated room.

And also the music style or the instruments to be recorded or mixed are decisive for the question of the absorber depth. If it is only a question of voice recordings and editing like it can be found in many YouTube studios nowadays, then absorption below 80 Hz is not relevant and thus a thin absorber is sufficient.

When the goal is defined, the question arises of how the room currently “looks” like and what problems it has. If there is a distinctive room mode at 40Hz that is critical to my music, then I have to make an absorption in the right place to make up for it. If, on the other hand, the room is reverberant but decays relatively evenly due to its spatial shape without overemphasizing individual frequencies, then the effort can be less.

And in addition to porous absorbers, there are of course other types such as membrane absorbers or Helmholtz absorbers. However, I think using porous materials like mineral wool is the easiest way to improve room acoustics.

Relationship between absorber depth and lower cut-off frequency

As a summary, let's take a look again at the flow resistivities mentioned above as a guide for the respective absorber depths:

Absorber Depth	Suitable Flow Resistivity	Rock wool	Glasswool	Caruso Iso Bond	Hemp
5cm (2")	30000 Pa*s/m²	70 kg/m³ (4.4 lbs/ft³)	55 kg/m³ (3.4 lbs/ft³)
5cm + 5cm gap	25000 Pa*s/m²	60 kg/m³ (3.8lbs/ft³)	48 kg/m³ (3.0 lbs/ft³)
10cm (4")	15000 Pa*s/m²	50 kg/m³ (3.1 lbs/ft³)	25 kg/m³ (1.6 lbs/ft³)
10cm + 5cm gap	14000 Pa*s/m²	50 kg/m³ (3.1 lbs/ft³)	24 kg/m³ (1.5 lbs/ft³)
20cm (8")	8000 Pa*s/m²	30 kg/m³ (1.9 lbs/ft³)	18 kg/m³ (1.1 lbs/ft³)	WLG 035
20cm + 10cm gap	6000 Pa*s/m²	25 kg/m³ (1.6 lbs/ft³)	16 kg/m³ (1.0 lbs/ft³)
30cm (12")	5000 Pa*s/m²		13 kg/m³ (0.8 lbs/ft³)	WLG 040
40cm (16")	3500 Pa*s/m²			WLG 045	35 kg/m³ (2.2 lbs/ft³)

If we adapt the flow resistivity to the absorber depth in this way, we see the linear relationship between the absorber depth and the lower end of the effective range. If you are wondering how deep your absorber should be, this is the graph that is most likely to give you an answer.

When it comes to speech or singing, you could make good improvements with 10cm. The human voice extends down to 100Hz and the 10cm are not yet ideal.

As soon as it comes to music with drums and bass, frequencies below 100 Hz will probably also play an important role. And an absorption in this frequency range can be achieved with 20cm.

What is the best material for me?

If, on the one hand, we now know how thick our absorber should be and then we use the example curves to read the area in which our flow resistivity should be, we can go to the last question about the material.

For the absorption properties it doesn't matter whether rock wool or glass wool or a special acoustic foam like Basotect or Caruso Iso Bond. You can use the material table at the beginning of this article to find some common types and approximate prices as a first orientation. With a little luck, you will find one of the fabrics in your local hardware store. But it doesn't have to be exactly the same type. Any other manufacturer can be used for glass wool and rock wool as long as the density (in kg / m³) is in the corresponding range.

I personally started my first attempts at building absorbers with rock wool because it is cheap and available everywhere. One disadvantage is certainly the health risk that you are exposed to during processing. If you approach the matter with suitable clothing, gloves and, if necessary, a respirator and wrap the rock wool in such a way that later no flakes can enter the room, then I think rock wool is a great material for acoustic purposes.

Glass wool should be very similar, although it is said to have even higher health risks.

On the one hand, it is really low-risk with natural products such as hemp or sheep's wool. As you can see in the table, the flow resistances are in the very low range, so that we can only use these two substances optimally for thick absorbers.

In its natural state, sheep's wool is even far below what we wanted to use for our purposes. By compressing more material into a smaller space, however, the flow resistance can be increased significantly.

Now that I have extensive experience with rock wool, my next level will be Caruso Iso Bond. The most important argument for this building material is certainly that it is less health-critical and cleaner to process than mineral wool. On the other hand, you get Caruso Iso Bond with all relevant densities and flow resistances, both for thick and thin absorbers. The only disadvantage: it is considerably more expensive than rock wool or glass wool.

[Addendum: You can find my comparison between Caruso Iso Bond and Rockwool here.]

From an acoustic point of view, most materials can be used. It is more a question of your wallet and your personal demand for a clean environment, whether you will be happy with rock wool, with hemp or with Caruso Iso Bond. Your ear will definitely be happy if you dedicate yourself to the topic of room acoustics and build your first absorber!

Blog article: Measurement microphone comparison test

Blog article: Caruso Iso Bond vs Rockwool - My reasons for a change

Blog article: How to measure room modes with REW (Freeware)